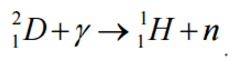Conservation of Energy in Nuclear Reactions
In analyzing nuclear reactions, we have to apply the general law of conservation of mass-energy. According to this law mass and energy are equivalent and convertible one into the other. It is one of the striking results of Einstein’s theory of relativity. This equivalence of the mass and energy is described by Einstein’s famous formula E = mc2.
Generally, in both chemical and nuclear reactions, some conversion between rest mass and energy occurs, so that the products generally have smaller or greater mass than the reactants.
Conservation of Energy in Nuclear Reactions
In general, the total (relativistic) energy must be conserved.
The “missing” rest mass must therefore reappear as kinetic energy released in the reaction. The difference is a measure of the nuclear binding energy which holds the nucleus together.
The nuclear binding energies are enormous, they are of the order of a million times greater than the electron binding energies of atoms.
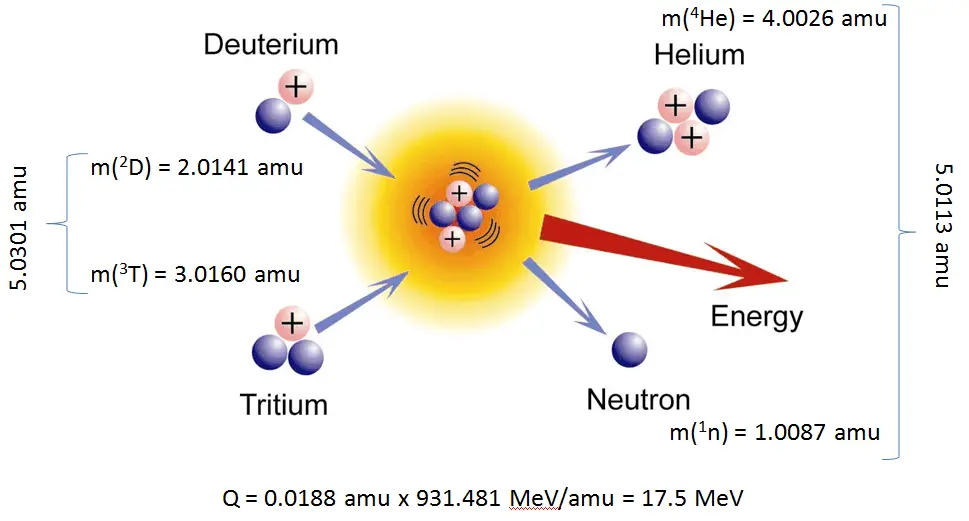
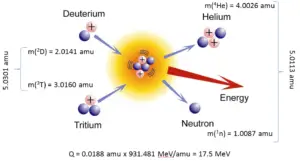
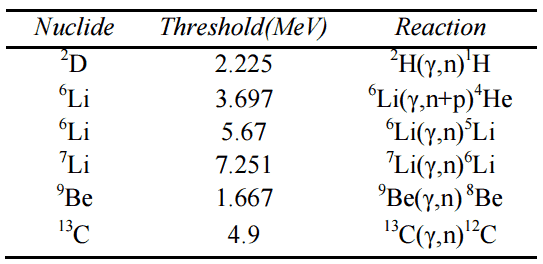
The energetics of nuclear reactions is determined by the Q-value of that reaction. The Q-value of the reaction is defined as the difference between the sum of the masses of the initial reactants and the sum of the masses of the final products, in energy units (usually in MeV).
Consider a typical reaction, in which the projectile a and the target A gives place to two products, B and b. This can also be expressed in the notation that we used so far, a + A → B + b, or even in a more compact notation, A(a,b)B.
See also: E=mc2
The Q-value of this reaction is given by:
Q = [ma + mA – (mb + mB)]c2
which is the same as the excess kinetic energy of the final products:
Q = Tfinal – Tinitial
= Tb + TB – (Ta + TA)
For reactions in which there is an increase in the kinetic energy of the products Q is positive. The positive Q reactions are said to be exothermic (or exergic). There is a net release of energy, since the kinetic energy of the final state is greater than the kinetic energy of the initial state.
For reactions in which there is a decrease in the kinetic energy of the products Q is negative. The negative Q reactions are said to be endothermic (or endoergic) and they require a net energy input.
See also: Q-value Calculator
The DT fusion reaction of deuterium and tritium is particularly interesting because of its potential of providing energy for the future. Calculate the reaction Q-value.
3T (d, n) 4He
The atom masses of the reactants and products are:
m(3T) = 3.0160 amu
m(2D) = 2.0141 amu
m(1n) = 1.0087 amu
m(4He) = 4.0026 amu
Using the mass-energy equivalence, we get the Q-value of this reaction as:
Q = {(3.0160+2.0141) [amu] – (1.0087+4.0026) [amu]} x 931.481 [MeV/amu]
= 0.0188 x 931.481 = 17.5 MeV
A high energy photon (gamma ray) can under certain conditions eject a neutron from a nucleus. It occurs when its energy exceeds the binding energy of the neutron in the nucleus. Most nuclei have binding energies in excess of 6 MeV, which is above the energy of most gamma rays from fission. On the other hand there are few nuclei with sufficiently low binding energy to be of practical interest. These are: 2D, 9Be, 6Li, 7Li and 13C. As can be seen from the table the lowest threshold have 9Be with 1.666 MeV and 2D with 2.226 MeV.
threshold energies.
In case of deuterium, neutrons can be produced by the interaction of gamma rays (with a minimum energy of 2.22 MeV) with deuterium:
The reaction Q-value is calculated below:
The atom masses of the reactant and products are:
m(2D) = 2.01363 amu
m(1n) = 1.00866 amu
m(1H) = 1.00728 amu
Using the mass-energy equivalence, we get the Q-value of this reaction as:
Q = {2.01363 [amu] – (1.00866+1.00728) [amu]} x 931.481 [MeV/amu]
= -0.00231 x 931.481 = -2.15 MeV
- Kinetic energy of the products
- Emission of gamma rays. Gamma rays are emitted by unstable nuclei in their transition from a high energy state to a lower state known as gamma decay.
- Metastable state. Some energy may remain in the nucleus, as a metastable energy level.
A small amount of energy may also emerge in the form of X-rays. Generally, products of nuclear reactions may have different atomic numbers, and thus the configuration of their electron shells is different in comparison with reactants. As the electrons rearrange themselves and drop to lower energy levels, internal transition X-rays (X-rays with precisely defined emission lines) may be emitted.
Energy Conservation in Nuclear Fission
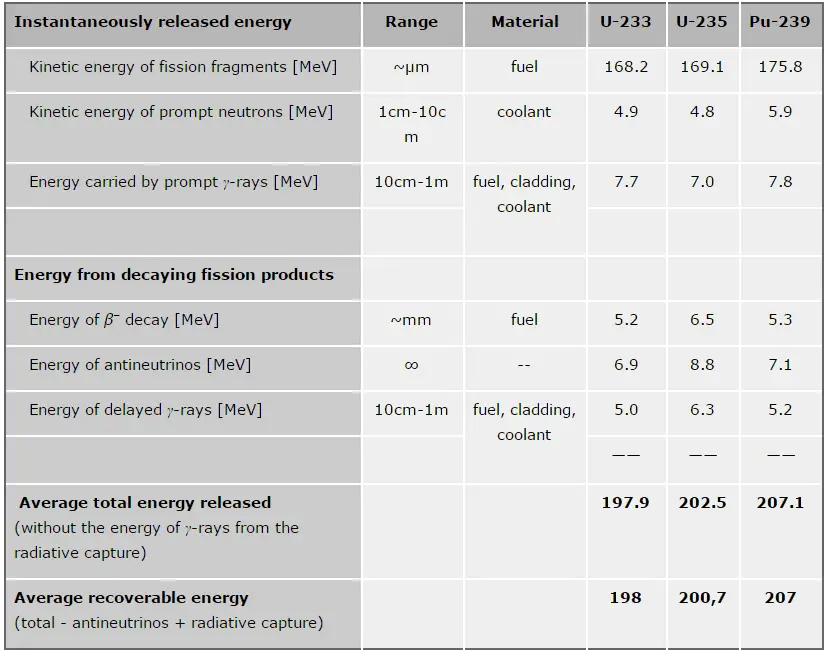In general, the nuclear fission results in the release of enormous quantities of energy. The amount of energy depends strongly on the nucleus to be fissioned and also depends strongly on the kinetic energy of an incident neutron. In order to calculate the power of a reactor, it is necessary to be able precisely identify the individual components of this energy. At first, it is important to distinguish between the total energy released and the energy that can be recovered in a reactor.
The total energy released in fission can be calculated from binding energies of initial target nucleus to be fissioned and binding energies of fission products. But not all the total energy can be recovered in a reactor. For example, about 10 MeV is released in the form of neutrinos (in fact antineutrinos). Since the neutrinos are weakly interacting (with extremely low cross-section of any interaction), they do not contribute to the energy that can be recovered in a reactor.
See also: Energy Release from Fission
Energy Conservation in Beta Decay – Discovery of the Neutrino
Beta decay (β-decay) is a type of radioactive decay in which a beta particle, and a respective neutrino are emitted from an atomic nucleus. Beta radiation consist of beta particles that are high-energy, high-speed electrons or positrons are emitted during beta decay. By beta decay emission, a neutron is transformed into a proton by the emission of an electron, or conversely a proton is converted into a neutron by emission of a positron, thus changing the nuclide type.
The study of beta decay provided the first physical evidence for the existence of the neutrino. This physical evidence is based on the law of conservation of energy during the process of beta decay.
In both alpha and gamma decay, the resulting particle (alpha particle or photon) has a narrow energy distribution, since the particle carries the energy from the difference between the initial and final nuclear states. For example, in case of alpha decay, when a parent nucleus breaks down spontaneously to yield a daughter nucleus and an alpha particle, the sum of the mass of the two products does not quite equal the mass of the original nucleus (see Mass Defect). As a result of the law of conservation of energy, this difference appears in the form of the kinetic energy of the alpha particle. Since the same particles appear as products at every breakdown of a particular parent nucleus, the mass-difference should always be the same, and the kinetic energy of the alpha particles should also always be the same. In other words, the beam of alpha particles should be monoenergetic.
It was expected that the same considerations would hold for a parent nucleus breaking down to a daughter nucleus and a beta particle. Because only the electron and the recoiling daughter nucleus were observed beta decay, the process was initially assumed to be a two body process, very much like alpha decay. It would seem reasonable to suppose that the beta particles would form also a monoenergetic beam.
To demonstrate energetics of two-body beta decay, consider the beta decay in which an electron is emitted and the parent nucleus is at rest, conservation of energy requires:
Since the electron is much lighter particle it was expected that it will carry away most of the released energy, which would have a unique value Te-.
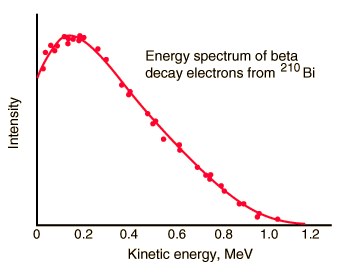
But the reality was different. The spectrum of beta particles measured by Lise Meitner and Otto Hahn in 1911 and by Jean Danysz in 1913 showed multiple lines on a diffuse background, however. Moreover virtually all of the emitted beta particles have energies below that predicted by energy conservation in two-body decays. The electrons emitted in beta decay have a continuous rather than a discrete spectrum appeared to contradict conservation of energy, under the then-current assumption that beta decay is the simple emission of an electron from a nucleus. When this was first observed, it appeared to threaten the survival of one of the most important conservation laws in physics!
To account for this energy release, Pauli proposed (in 1931) that there was emitted in the decay process another particle, later named by Fermi the neutrino. It was clear, this particle must be highly penetrating and that the conservation of electric charge requires the neutrino to be electrically neutral. This would explain why it was so hard to detect this particle. The term neutrino comes from Italian meaning “little neutral one” and neutrinos are denoted by the Greek letter ν (nu). In the process of beta decay the neutrino carries the missing energy and also in this process the law of conservation of energy remains valid.
We hope, this article, Conservation of Energy in Nuclear Reactions, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about materials and their properties.